How Does Social Security Know How Much You Make in Your 66th Birthday
The Birthday Trouble🎈
Today'southward problem goes out to a special new member of the family. Welcome to the world my niece, Edison Grace Berry! My brother's beautiful baby girl was born on his 36th birthday this by Saturday and of course this coincidence fabricated me recall of the Birthday Problem .
So hither it is: a special math trouble for a special niggling girl. Someday you'll know all the math to understand this mail service (trust me, I'll make certain of it!).
→ For more math tutorials, cheque out Math Hacks on YouTube! ←
The Birthday Trouble in Real Life
The first time I heard this trouble, I was sitting in a 300 level Mathematical Statistics class in a small academy in the pacific northwest. It was a grade of about 30 students and the professor bet that at to the lowest degree two of the states shared the same birthday.
He then proceeded to have anybody country their birthday. When it came to my turn I stated my birthdate as "two cubed, 3 cubed," which made the grade laugh as our cerebral professor took awhile to decipher the appointment.
Anyway like he predicted before he got to the concluding pupil a pair of matching birthdays had been plant.
So how lucky was it that he found a matching pair?
Warm-Up
Assumption: for the sake of simplicity we'll ignore the possibility of being built-in on Feb. 29th.
Let'due south begin with a simple example to warm upward our brains:
What is the probability that two people share the same birthday?
Person A can be born on whatsoever day of the twelvemonth since they're the first person we're asking. The probability of being born whatever day of the year is i or more specifically: 365/365.
Since Person B must be built-in on the same day as Person A their probability is 1/365.
We want both of these events to happen and then multiply the probabilities:

And so you take a 0.27% chance of walking upwardly to a stranger and discovering that their birthday is the aforementioned day every bit yours. That'southward pretty slim.
Just what virtually a larger group?
What's the chance that at least 2 out of 4 people share the aforementioned altogether?
Well to solve this problem nosotros'd have to calculate all of the following:
- Probability A and B share the same birthday
- Probability A and C share the aforementioned birthday
- Probability A and D share the same birthday
- Probability B and C share the same birthday
- Probability B and D share the same birthday
- Probability C and D share the aforementioned altogether
- Probability A, B and C share the aforementioned birthday
- Probability B, C and D share the same birthday
- Probability A, C and D share the aforementioned birthday
- Probability A, B and D share the same birthday
- Probability A, B, C and D all share the same altogether
Yuck, that's a lot of calculations! Imagine how many probabilities we'd have to calculate for a classroom of 30 students!
There'south gotta be a ameliorate style…
A Amend Way: the Trick of the Complement
The simplest style of getting around computing a bajillion probabilities is to look at the problem from a dissimilar angle:
What's the probability that no ane shares the same birthday?
This alternate practise is helpful considering it is the complete opposite of our original problem (i.eastward. the complement). In probability, we know that the total of all the possible outcomes (i.e. the sample space) is ever equal to 1, or 100% chance.
Since the probability of at least 2 people having the same altogether and the probability of no one having the same altogether embrace all possible scenarios, we know that the sum of their probabilities is 1.
Or equivalently:

Yay! That'll be much easier to calculate.
The Adding
Awesome! We're finally ready to find out how safe a bet the professor made.
Let's work out the probability that no ane shares the same birthday out of a room of 30 people.
Permit's take this stride by step:
- The offset student tin can be born on whatsoever day, and so we'll requite him a probability of 365/365.
- The next student is now limited to 364 possible days, so the 2d student's probability is 364/365.
- The third student may be built-in on whatsoever of the remaining 363 days, and then 363/365.
This pattern continues so that our final student has a probability of 336/365 (365 – 29 days since the students earlier her used upwards 29 potential days).
Once again multiply all 30 probabilities together:
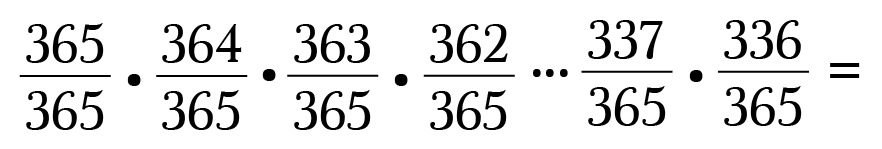
Hold up! That's a little messy. Let'southward clean this up.
Since the denominator is thirty 365's multiplied together, nosotros could rewrite it as:

Permit'southward use factorials (symbolically: !) to further clean this calculation upwards.
(Retrieve factorials are handy for multiplying together descending positive integers. For example 5! equals five•iv•3•ii•1 = 120.)
Using factorials, 365! would equal the product of all descending integers from 365 down to i. We merely want the production of the integers from 365 to 336, and then we'll dissever out the extraneous numbers by dividing 365! past 335!.

Note: if this confuses you endeavor a smaller value similar 5!/3! = 5•4•3•2•i / 3•2•1. Notice how the 3•2•ane are in both the numerator and denominator. They 'cancel out' making 5!/three! = five•4.
Putting information technology all together we now accept an expression that tin exist easily entered on a scientific calculator:

This computes to 0.294 or 29.4% gamble no one in the grade has the same birthday. Of course, we want the complement so we'll decrease it from one to detect the probability that at least 2 people in a group of 30 share the same 24-hour interval of birth.

Turns out it was a pretty condom bet for our professor! He had a nearly 71% run a risk that ii or more of u.s.a. would share a birthday.
A 50-Fifty Chance
Many people are surprised to find that if yous repeat this calculation with a group of 23 people you lot'll nevertheless take a 50% chance that at least two people were born on the same twenty-four hour period.
That's a relatively minor group of people considering that there are 365 possible birthdays! Pregnant that in whatsoever grouping of more than 23 people it is probable that at least 2 people share the same day of birth.
What a crazy little factoid!
❤ STAY Connected ❤
Stay up-to-engagement with everything Math Hacks is up to!
Instagram | Facebook | Twitter
Related Reading
Source: https://medium.com/i-math/the-birthday-problem-307f31a9ac6f
0 Response to "How Does Social Security Know How Much You Make in Your 66th Birthday"
Post a Comment